1. Ships
steel which is used to make ships is 6-7 times dense than water but a ship is able
to float on water because it is designed to displace more water than its volume. Load
lines called Plimsoll marks are marked on the side to indicate the maximum load at
different seasons to avoid overloading.
2. Submarines
they are made of steel and consists of ballast tanks which contain water
when theyhave to sink and filled with air when they have to float. This makes the
submarines to balance their weight and be able to rise upwards.
3. Balloons
when they are filled with helium gas balloons become lighter and the
upthrust on the balloon becomes greater than their weight therefore becoming able to
rise upwards.
4. Hydrometers
they are used to measure the relative densities of liquids quickly and
conveniently. Various types of hydrometers are made to measure different ranges of
different densities i.e. lactometer – for measuring milk water (range 1.015 – 1.045),
battery acid tester – used to test the charge in a lead-acid battery.
Examples
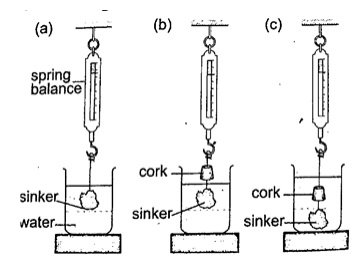
1. A solid of mass 1.0 kg is suspended using a thread and then submerged in water. If the
tension on the thread is 5.0 N, determine the relative density of the solid.
Solution
Mass of solid = 1.0 kg
Weight of solid W = mg = 10 N
Tension on the string (T) = 5 N
Upthrust on solid (u) = W – T = 10 – 5 = 5
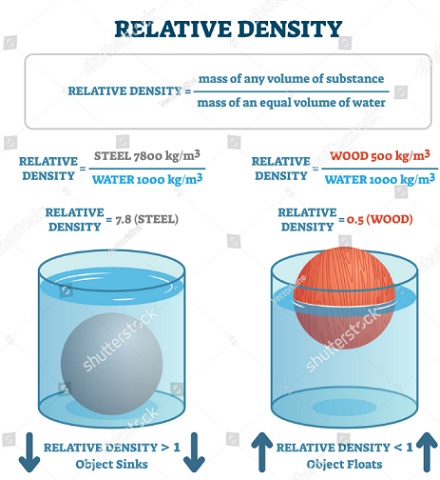
Relative density (d) = W / u = 10 / 5 = 2.
2. A balloon made up of a fabric weighing 80 N has a volume of 1.0 × 107 cm. the balloon is filled with hydrogen of density 0.9 kgm-3
Calculate the greatest weight in addition to that of the hydrogen and the fabric, which the balloon can carry in air of average density 1.25 kgm-3
Solution
Upthrust = weight of the air displaced
= volume of air × density × gravity
= (1.0 × 107 × 106 ) × (1.25 × 10)
= 10 × 1.25 × 10 = 125 N
Weight of hydrogen = 10 × 0.09 × 10 = 9 N
Total weight of hydrogen and fabric = 80 + 9 = 89 N
Total additional weight to be lifted = 125 – 89 = 36 N.
3. A material of density 8.5 gcm,-3
is attached to a piece of wood of mass 100g and density 0.2 gcm-3
Calculate the volume of material X which must be attached to the piece of wood so that the two just submerge beneath a liquid of density 1.2 gcm-3
Solution
Let the volume of the material be V cm
3
The mass of the material be 8.5 V grams
Volume of wood = 100 g / 0.2 g/cm = 500 cm
3
In order to have an average density of 1.2 gcm
-3
= total mass / total volume
Therefore (100 + 8.5V) / (500 + V) = 1.2 gcm
-3
Hence V = 68.5 cm
3